Hefty de Sitter charges, non-local divergences from reductions(!) and Final Day Sentiments! Adieu.

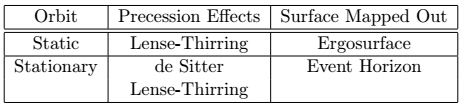
On the final day of ST4 2018, we begin with a bunch of thank yous. We are deeply grateful to Prof. Sudhakar Panda, the Director of NISER, for signing off on letting us have the second edition of ST4 here. Our sincerest and most heartfelt thanks to Prof. Chethan Gowdigere for his pivotal support in the organisation of resources for ST4 2018, without which (it is safe to say) the organisers would have had to struggle quite a bit. Thanks also to the staff at NISER and the chai & coffee supplying bhaiyyas who made sure we never ran out of little things to eat or drink during lectures.

We thank all the attendees for participating in the democratic process of picking our speakers, and in doing so, also choosing the theme for ST4 2018. Thanks also for attending and having a good time, and for making our little meeting a success! Finally, thanks to the speakers who were all wonderful, solely responsible for making this ST4 a lot of fun, and were fully prepared with a nifty answer against our every worry in the classroom– we learnt a lot!

We hope to see you all again soon, at ST4 2019!

*******

Jahanur came back on the last day of ST4 2018 to compute the energy in the de Sitter spacetime, using notions of symplectic structures introduced by Aneesh the previous day.

A brief review in possibly more familiar language follows. Consider the variation of the Langrangian under fields ,

where the last term is the total derivative whose integral variation we all like to set to zero on the boundary while deriving the equations of motion, which are denoted here by . Now, suppose the space of on-shell solutions has many parameters , which we can continuously tune to get from one to another solution of . Since is like a density, we would like to have . If we define,

then . Or more precisely, if we consider the vanishing of the action instead of then,

or, using Gauss’ Theorem,

If the above summary didn’t resonate deeply with you, you can always visit Aneesh’s lecture notes.

Now, since Jahanur wants to compute the energy of the system, he considers a special class of hypersurfaces which are those generated by the the time-like Killing vector of the de Sitter metric , and he defines the total energy to be on this surface. Notice that this wont simply vanish. It would have been zero if one added up the contribution from the other surfaces enclosing the system; this particular surface however, having been generated by time-like Killing isometry of the system, is designed to measure the energy escaping it.

This surface is defined by const. The for this case is given by,

where,

For the energy computation we take, (where is the Lie derivative w.r.t. ).

Diffeomorphisms are symmetries of any sane theory of gravity, and therefore the Lie derivatives of are also solutions to the linearized solutions. Next we fix a gauge by demanding that,

Anticipating algebraic convenience, Jahanur chooses to work with . These gauge conditions further restrict the set of allowed diffeomorphisms. We would like to find all invariant components of under this restricted set of diffeomorphisms. It turns out that the transverse traceless (TT) component of remains invariant. Given a , extracting the TT component is non-trivial since one needs to know the behaviour of the field as it approaches the boundary.

In usual practice (for flat spacetimes), according to the speaker, one gets by the above difficulty of computing the transverse traceless component by computing it only asymptotically. This is done by means of a propagator,

It turns out that,

That is, for flat space times, this difference in the two definitions does not matter as long as one is computing energy flux. Although, it may turn out to be important when one tries to compute angular momentum flux etc.

However, the above difference gives different notions of energy for de Sitter spacetimes. We compute this energy for .

where the tt indices are suppressed and .

where the constant surface is . Upon neglecting some total derivatives and assuming the ‘staticity condition’ at future and past infinity for the source, this can be written as,

where

Just to remind the reader,

which is the quadrupole moment in the de Sitter background.

***

We commenced after the break with Jahanur speaking to us about the ADM energy for de Sitter spacetimes,

where for being space/time-like respectively.

For the de Sitter metric,

we get . Now, let’s take the boundary of this de Sitter spacetime to be the two space like boundaries at large initial and final times and a time-like surface connecting these two space-like surfaces.

Forget about the term for a bit.

Now one has to juggle around with the (in)famous Gauss-Codazzi equations to rewrite in terms of and . The expression for the action reduces to,

where is normal to the timelike boundary surface. Further, , decomposing the metric on the spacelike cross-section as . Reminder: and . Representing and assuming the equations of motion to hold (enabling us to drop the bulk term) we get,

where the ADM mass is given by

Let’s look at some examples.

Computed for the flat Minkowski spacetime, this turns out to be,

where is the respective connection w.r.t .

Consider the Schwarzchild spacetime:

where is a constant-t surface. . When the dust settles one gets,

For de Sitter-Schwarzchild, this formula doesn’t work because does not have a good limit as .

The ADM mass can also be derived from the construction of a Noether current. (An astute reader might ponder how one should use Noether’s theorem in a theory which has only gauge symmetries. In fact, Noether’s second theorem deals precisely with gauge symmetries! :P).

where is the asymptotic time translation .

Jahanur also discussed Kelly & Marolf‘s results on de Sitter charges on flat and hyperbolic slices (for and respectively).

There is yet another way to compute asymptotic charges. This one seems to be in vogue in the AdS/CFT community. Here, one computes first what is known as the Brown-York stress tensor for an action. This basically is the variation of the Einstein-Hilbert action w.r.t the boundary metric. One chooses an appropriate slicing: for negative curvature spaces, the slicing should be time-like whereas for positive curvature spaces, it must be space-like. Then one computes the charge along ones favourite symmetry of the boundary metric in the usual sense, from this Brown-York tensor. For the de Sitter case

where

for the spacelike boundary surface.

Strominger et al., computed the charges for deSitter space. BUT! the asymptotic symmetry group of deSitter (according to Jahanur) is . How then does one single out a physical subset? Ashtekar proposes to compute this charge on the cosmological horizon , imposing the condition of no incoming radiation.

With this set of final comments, Jahanur bowed out. Phew, that was hectic and informative!

*******

Chandan in his last lecture discussed open theory. He first introduced the action for this theory and read off the Feynman rules for various propagators and vertices. He pointed out that in closed (unitary) Schwinger-Keldish (SK) theory left and right fields do not mix at the level of action and hence mixed interaction vertices having both left and right fields never appear. This is not true in case of open quantum theories, and mixed vertices do appear.

He then continued to discussed the renormalisability of this theory. By considering various beta functions for the couplings, he showed us how under renormalisation group flow the tree-level Lindblad conditions are protected even at one loop. He further mentioned that the Lindblad conditions are actually all loop exact! This strongly implies that

Markovianity of open theory is preserved under renormalisation.

To calculate the beta functions for the quartic couplings at one loop, divergent contributions come from bubble diagrams. All the diagrams can be computed by evaluating just a single integral over loop momentum. This integral is hard to compute since space and time can not be treated on equal footing due to the structure of the integrand. With some hard work the integral can be performed to obtain all the beta functions till quartic order in the couplings, at one loop.

Chandan then discussed various non-local divergences that appear in the loop integrals for open quantum field theories by considering the case of theory. These non-local divergences are inherent to open quantum field theories seemingly due to the fact that quantum field theories have infinite number of degrees of freedom as compared to any quantum mechanical system.

As an example consider the following amputated diagram (Fig 1) in theory with the masses and of the two propagators in the loop.

Fig 1

The integral evaluates to,

where k is the external momentum. Notice that there is a in the denominator of the above expression, making it nonlocal. There is an infrared (IR) divergence for small k as well as an ultraviolet (UV) divergence for . The non-local divergence disappears for .

The second example (Fig 2) to illustrate nonlocal divergence was for the Yukawa theory. Chandan considered the following self energy diagram for Dirac fermion.

Fig 2

 

Using the Passarino Veltman (PV) reduction, one can reduce the fermionic integral into a linear combination of two types (called the A and the B type) of scalar loop integrals. These integrals have non local divergences that prevail even when one sets . The physical origin of such non local divergences is not well understood. With a review of more such intriguing currently open questions, Chandan signed off.

And that was that. The organisers announced that ST4 2018 was at a close. Murmurs of the sort “okay, where do I sign up for ST4 2019” were heard and everyone happily left the classroom to go pack.

*******

Goodbye and thanks for following ST4 again this year.

See you again in 2019!

We will be back.

Presymplectic Flows, Lindblad the Sailor and Hot, Hot CFTs!

Today’s lecture, by Aneesh P B (CMI, Chennai), was focused on introducing the covariant phase space formalism with a view toward its use in calculating charges in de Sitter.

The phase space of a classical system is usually introduced as the space of positions and momenta of the system at a particular instant in time. However, we can think of each point in this space, given by a position and a momentum , as an initial condition for classical evolution and therefore the phase space is also the space of classical solutions.

The point of this reformulation is that while the first point of view depends on the picking of a time-slice and therefore breaks (local) boost symmetry, the second formulation doesn’t care about this. Because gravity is invariant under diffeomorphisms and therefore under local boosts, this covariant point-of-view of phase space is more natural.

The main problem with this point-of-view is how to calculate the symplectic form (the inverse of which is related to poisson brackets), which is required (among other things) for calculating dynamics. There are multiple approaches to this, for example the one by Crnkovic and Witten and the one by Lee and Wald. Aneesh followed the Lee and Wald formalism.

Aneesh began with a short introduction to forms and differential geometry. For those who need a refresher: a -form is a fully antisymmetric tensor with lower indices, and it’s written as,

where denotes wedge product which is just a fancy way of saying that the different s anticommute with each other.

Also important is the exterior derivative, which is a fully antisymmetrised derivative of a form, so that the final result is a -form. The reason forms are interesting is that they are the natural objects that are integrated on -dimensional surfaces — the antisymmetrisation is so that the integral gives zero when two of the ‘s point in the same direction; this is why the volume of a parallelopiped is the fully anti-symmetrised product of its three ‘basis’ vectors.

He then introduced the symplectic form as a two-form on phase space. For example, in an n-particle case, it is,

where is a way to label both positions and momenta with the same variable, so that . The Poisson brackets are given by the inverse of the symplectic form,

Then, he introduced the concepts of ‘Hamiltonian vector fields.’ Consider a vector field on phase space, . These can be thought of as flows on phase space, by identifying the components with the rate of variation of the coordinates, (where this derivative need not be with actual time, just come continuous parameter that parametrises the amount of flow). There are two types of flows on phase space: those that can be written as

and those that can’t be. Clearly, for consistency with Hamilton’s equations, we need time evolution to be of the first form. One might also want symmetry transformations to be of this form, for example. In a massive failure of sense and sensibility in nomenclature, flows that can be written as the Poisson brackets of a function on phase space with the coordinates are called ‘Hamiltonian vector fields’ and the functions whose Poisson brackets they are are called the Hamiltonian conjugate to : the Hamiltonian is the hamiltonian conjugate to time translation, the momentum is the hamiltonian conjugate to space translation, and in general a Noether charge is the Hamiltonian conjugate to a symmetry (up to possible subtleties).

An important question that would be required in the gravity case was: given a flow, how does one tell whether it is Hamiltonian or not? The answer is that the “Lie derivative of the symplectic form” along this vector should be zero. The Lie derivative of a tensor along a vector is the formalisation of the way the tensor changes under the flow corresponding to that vector, and this condition ensures that the symplectic form is invariant under this flow. In particular, the Hamiltonian conjugate to such a flow is the conserved quantity of that transformation of the phase space coordinates.

Moving to gravity in dimensions, he began with the space of all ‘kinematically allowed’ metrics on a manifold , where by ‘kinematically allowed’ he meant metrics one wouldn’t be devastated to find as solutions to one’s equations of motion. He then explained the rather involved process of defining the symplectic form as a two-form on the manifold (note that there are now two manifolds, the spacetime and the space of metrics — we will have to be careful about where we’re differentiating).

The process is simple but unintuitive. First, take the Lagrangian , which maps every point in to a -form on the spacetime ; this -form is just the scalar Lagrangian we normal human beings are used to, multiplied by the and the completely antisymmetric -index Levi-Civita tensor. Then, vary it, getting something of the form

Here, are the Einstein equations, and the second term is the familiar boundary term one always finds (and willy-nilly sets to 0) while integrating by parts to find equations of motion from a Lagrangian — in this case, it is known as the presymplectic potential. The name is analogous to a gauge potential, since and are indistinguishable — there is a gauge-invariance in , and changing gauge will correspond to canonical transformations on the phase space (which hasn’t been defined yet!). Finally, the presymplectic current is defined as the -form,

and the presymplectic form can be found by integrating the presymplectic current over a -dimensional surface — for example, the surface on which one would like to specify initial data. The prefix pre to all the symplectic objects are because they are not true symplectic objects – the reason being that they are highly degenerate (along the gauge directions) on the covariant phase space. The reason we went through all this was that

1. we didn’t need to specify the intial data surface till the very end, so it can be anything as long as it is a valid initial data surface.
2. This symplectic current is a conserved current, in the sense that its integral on a closed surface is 0, because of which it is the same for all spacelike surfaces that can be deformed to each other.

However, we still weren’t done: this symplectic form wasn’t defined on the phase space but on the space of all “kinematically allowed” metrics! So, we had to restrict from to the space of solutions . Then, we got the real pre-symplectic form. This was still not the true phase space, since two diffeomorphism-related metrics are two different points in despite being the same physical state, but it turned out that there were some subtleties too subtle even for our brave knight, and this was one of them.

From here, the process for calculating the charge is simple, up to some boundary term issues. Plug in a particular value for say in the symplectic form to get the generator of infinitesimal transformations of that form, and then try to integrate it to find the Noether charge. He showed how this worked for the specific example of the charge conjugate to the translations in conformal time that Jahanur had indicated would be central to understanding gravitational waves in de Sitter.

*******

Chandan began his third lecture with the derivation of the Lindblad equation, starting from the basic assumption of Markovianity which he had covered in the second lecture. He did this by starting with the equation,

which was shown to follow from Markovianity in the previous lecture, where

This time, we expanded in terms of number of orthonormal basis matrices . We then took a particular basis set in which and all other basis matrices are traceless (For eg. form a basis of complex matrices). We then defined some new variables in terms of these basis matrices. The condition that the evolution preserves the trace, was the final ingredient to derive the Lindblad equation.

where was shown to be a positive semi definite matrix. Curious readers can access the handwritten notes at the resources section of the ST4 2018 website. At this point, a discussion arose as to how the trace preserving condition was used, where it was also pointed out that we had used . The fact that commutator has zero trace while true for finite dimensional systems (as ), is not true for infinite dimensional systems in general. We then wondered if this told us that Lindblad equation applied only to a finite dimensional Hilbert space, or if the particular trace we evaluated would still be zero by some nice properties of the Hamiltonian and the density operator. In any case, the audience, who struggled to comprehend the jump to the Lindblad equation from the assumptions on the second day, were happier when this outline was presented and were ready for new stuff.

Chandan then introduced Veltman’s cutting rules to diagnose whether a theory is unitary. Consider the scattering matrix of a unitary quantum field theory. Then . Define such that , then the unitarity condition can be written as,

where are some basis vectors. Chandan told us that (according to Veltmann), this should be true diagram by diagram. It was pointed out by members in the audience that (if we trust Veltmann) in a theory, if we apply this to the four point function on the left side, we obtain product of three point vertices on the right side which are zero. Hence, in this case, the condition reduces to the imaginary part of being zero. Thus must be real in order to have a unitary quantum field theory.

We then studied the cutting rules in Schwinger Keldysh. Chandan reminded us from the first lecture that for , and . This was recast as the largest time equation for general diagrams. We used the largest time equation to “cut” various diagrams, in the sense that the RHS, and are on-shell. Therefore, the largest time equation can be used to make the internal lines (off-shell) on the left side to external lines (on-shell) in the diagrams appearing on the right side, which can also be seen as cutting the diagram on the left in various ways to convert internal lines to external lines.

Chandan then showed us how if we define a different basis (called the average-difference basis) in the Schwinger-Keldysh space , then the cutting equation reduces to the statement that all correlation functions with the difference field are zero.

*******

The last in the long line of evening lectures was presented by Kausik Ghosh (IISc, Bengaluru). He spoke to us about CFT bootstrapping at finite temperature. He started with a remark on how conformal invariance fixes all one-point functions of any operator in a CFT on to zero, barring the identity operator. Now, he argued, we can compactify one of the direction and study the CFT on a , where the length of the circle can be identified as a temperature. But introducing a length scale in the system will cost us: we will now have nonzero one-point functions for arbitrary primary operators, in principle. Although, because of the translational invariance, one-point functions of any descendents will still vanish. The one point function of a scalar primary operator is given by,

Kausik then went on to explain how symmetries restrict one-point functions of operators with spin as well. Now, a key point: if the distance between two operators is less than the length of the circle, we can use the operator product expansion of two operators (same as zero temperature CFT). Doing this repeatedly reduces any n-point function to one-point functions, which are in turn fixed in terms of , he says. Using OPE, a two-point function can be written as,

where and . is the full conformal block for a thermal two point function.

Here is the coordinate along the circle and $x$ is in $R^{d-1}$. The expression for the coefficient is,

These crossing relations for two-point functions (called the KMS relations) are due to the periodicity along the direction, which are like the crossing symmetry relations of conformal blocks. But since the sign of is not fixed, one cannot directly apply numerical bootstrap techniques. Rather, we will derive a powerful inversion formula which will enable the use of the KMS condition to do large spin analysis.

We can complexify and the two-point function can be written as,

The operators have simple poles in the space of s and have residues in ,

We use the inversion formula for to find the expression of , using some properties of Gegenbauer polynomials and a Laplace transform. The integration is in Euclidean space. invariance allows us to fix all the coordinates along a line and measure distance only in that direction. Therefore, we use coordinates and . For simplicity, Kausik showed the derivation of inversion formula in 2d, but similar reasoning follows through in higher dimension.

The Gegenbauer polynomials for 2-dimensions are given as,

where and . So, in the Euclidean version the formula for looks like,

We assume that the two-point function is analytic away from brunch cuts , and in the complex plane. Then, Kausik explained that the contour can be deformed around the bruch cuts. For our purpose, we deform the contour for towards the origin and for , we deform the contour towards the infinity. There is a symmetry under , which relates these deformations.

Assuming a particular fall off for two-point functions towards large , he showed that we can write down the final form of inversion formula for in terms of only the discontinuity of the two point function. While deforming the contour towards infinity, what we actually did is we analytically continued therefore obtaining the Lorentzian inversion formula, starting from a Euclidean formula.

Finally Kausik motivates us with some examples and future directions to explore. He mentions that this type of analysis usually used in mean field theory where main interest is to study critical points at finite temperature. For critical type model in large , the expectation value of the field involved gives the thermal mass . Numerically, the thermal mass has been solved for. In the finite temperature regime, the correlators of energy momentum tensors are not studied extensively. It will help to explore the AdS side and to also find transport coefficients.

After this, due to the shortage of time Kausik briefly outlined how large spin perturbation theory works in this setting. Also how large spin resummation can generate poles for other operators present in theory and how does this gives correction to pole locations.

With Kausik signing off, another meeting is almost at a close. Just a couple of lectures remain and by this time tomorrow, we’ll be looking towards ST4 2019!

Foliations, LCRs and Precession

Jahanur started off his second lecture with the aim of reviewing the Hamiltonian formalism of GR. The formalism was developed for a four-dimensional manifold . The speaker announced at the start that he would not distinguish between the indices of and those of the metric over hypersurfaces with no timelike coordinate (which we shall shortly introduce) and thus, running over three possible values. This spacetime can be foliated using non-intersecting spacelike hypersurfaces with , the coordinate along the timelike direction labelling these various hypersurfaces.
Defining the future directed normal as,

we can write the induced metric on the spatial hypersurface as

and the raised projection tensor is, . Note that is not the inverse metric. The speaker pointed out that all indices were raised/lowered with respect to , the four-dimensional metric on the full manifold. can be thought of as a projection tensor which projects out all geometric objects lying along . Following the construction of projection operators and studying their action on vectors and tensors, Jahanur proceeded to define covariant differentiation of a “purely spatial” vector field , restricted to the hypersurface . Predictably, it is essentially the projection of the four-dimensional its covariant derivative on the hypersurface i. e.,

For “purely spatial tensors,” an analog of the above equation with more s sitting in front would be the appropriate definition of the covariant derivative on . For general tensors, with a non-zero projection along the normal, simply considering the covariant derivative and projecting it on won’t give you a purely spatial derivative: it will contain a piece proportional to the extrinsic curvature. The Riemann tensor for the hypersurface has no information about curvature of the embedding space . This is captured through the extrinsic curvature which is defined as,

which is symmetric in . Through a series of manipulations and making use of the explicit form of , Jahanur eventually showed that the extrinsic curvature tensor is related to the Lie derivative of the induced metric along the normal vector, namely

After the coffee break, in an attempt to relate the Riemann tensor associated with in terms of the the Riemann tensor for , Jahanur ended up with the Gauss-Codazzi equations:

These are essentially integrability criteria which specify a particular spatial hypersurface through the data in a higher dimensional ambient spacetime. These equations are also crucial in decomposing Einstein’s Equations in the Hamiltonian formulation which gives us the Hamiltonian constraint and the momentum constraint. They take the following form:

These equations capture the dynamics of a gravitational field at a particular snapshot of time const.

The most natural question to ask after this point is what about the evolution of the data as we go from one hypersurface to . It is to be noticed here that the Lie derivative along is not a natural time derivative thus necessitating the construction for . Starting with a one-form, which is normalized as . We define another vector , where is a spatial shift vector (thus, is dual to the vector). Physically, the vector is the congruence which takes a small patch from the hypersurface to another hypersurface infinitesimally away . The vector captures the shift in the coordinate points with respect to the normal. This spurred off an enthusiatic discussion amongst the audience regarding the special case when the vector is vanishing. Eventually, Jahanur wrote down the explicit form of in terms of derivatives of and and their derivatives.

The formalism building was followed by the discussion of a special case where , which is true if we make the choice . Over the last quarter of an hour, Jahanur described the ADM formalism starting from the Einstein-Hilbert action. He wrote down the Lagrangian density in terms of the hypersurface variables and gave a nice compact expression for the conjugate momenta on as,

In the last few minutes, he defined the electric part of the Weyl tensor in the context of de Sitter spaces with the promise that Aneesh would pick up from here and explain how it comes about in the next lecture. With hunger in our minds and stomach, we proceeded for lunch.

*******

After lunch, the inimitable Chandan Kumar Jana began his second lecture on open quantum systems, with the goal of introducing dissipation, defining notions of open quantum mechanics, and deriving the Lindblad equation. Historians of science will remember this evening as an especially entertaining one.

Before getting on with the days proceedings, Chandan started out by highlighting that the usual Schwinger-Keldysh contours are inadequate for computing certain correlators. Consider, as an example, the following correlator

with the time ordering and . A little fiddling around with contours will convince one that the last operator cannot be accommodated with the constraint ! This can be remedied in a straightforward manner: introduce another time-fold. In general, a -out of time ordered correlator (OTOC) is one with future turning points. For example, a -OTOC is the usual Schwinger-Keldysh contour, and a -OTOC is typically used in the computation of the chaos correlator.

We then moved on to business for the day. Recall from our example yesterday — the one with the particle attached to a spring that was in turn attached to a pendulum — that we were able to integrate out the position of the spring/oscillator, , to write down an effective action for the position of the particle (tip of the pendulum) denoted by that looked (schematically) like this:

where is the usual Schwinger-Keldysh action, and is the Feynman-Vernon influence functional, which has a complicated form and has information regarding the variable that has been integrated out. It is important to note, however, that the influence functional (written below in frequency space) itself has interesting structure:

The quantity , defined as,

is an impedance, Chandan said, and the room fell silent. “What the hell did impedance have to do with anything?,” some members of the audience members wondered. Then Chandan muttered the words “LCR circuit,” and all hell broke loose.

Perhaps some explanation is in order: although it is actually not at all difficult to work out, the mention of certain topics — usually those from undergraduate textbooks — like Carnot engines, for example, send the otherwise respectable graduate student into a panic-induced fury. This was one of those times. We had, however, the dulcet tones of Prashant Kocherlakota (TIFR), who helpfully reminded the audience what an LCR circuit is. Basically, if a circuit has an inductor with inductance , a capacitor with capacitance , and a resistance with resistance , all in series, the equation obeyed by the charge as a function of time is,

where is the potential and plays the role of the forcing term in an LCR circuit. The above equation is simply solved in the frequency domain by,

which contains essentially the Fourier transform of the Green’s function. Basically, if the Green’s function has an imaginary part, you’re going to have dissipation. In the case of the particle , it’s going to give some of its energy to the oscillator that we’d integrated out, and in the case of an LCR circuit, it is going to leak out because current flowing through a resistor will heat it up.

On to open quantum mechanics. We assume that the Hilbert space of our total system splits into two parts: that of the subsystem we’re interested in and that of the environment . The strategy of our computation will be to start with a density matrix ,

where is not a density matrix; rather, it keeps track of all correlations between the system and its environment, a sort of correction term. We want to start with this state and evolve in time to , then trace out the environmental degrees of freedom. This is a bit involved, so we’ll save the details for the notes and state the end result here:

where are called Kraus operators, and are essentially matrix elements of the global unitary time evolution operator in some environmental basis. Chandan then stated a couple of theorems without proof:

•  if the initial -state is pure, then is zero identically, and
• the above equation for can re-written by redefining the Kraus operators to depend on the initial -state, and this has the effect of absorbing the .

We’ll end our discussion of Chandan’s lecture for today by discussing a particularly thorny issue regarding the manner in which quantum systems evolve. Theorem 1 above states that if we start off with a pure state, then is identically zero. Now consider time-evolving a system from to in two different ways: first, directly; and second, by stopping off at first. Define a universal dynamical map (UDM) as one where the Kraus operator doesn’t depend on the initial -state, and a Markovian evolution as one where time-evolution is derived by composing UDMs. As we have seen, however, this can’t work because in the time between and , the system will have developed correlations, and consequently will not be a pure state.

In general, open quantum system evolution is not Markovian. However, in those situations where doesn’t affect the dynamics appreciably, i.e., when the time scale for decay of system-environment correlations is smaller than the time scale on which we are interested in tracking (or probing) the evolution of the system, we can make a Markovian approximation. Assuming this, we can derive a linear time-evolution equation called the Lindblad equation, whose details we will explore in greater detail tomorrow.

*******

The evening talk of the day was delivered by Prashant Kocherlakota (TIFR, Mumbai) on the time evolution of spins in a gravitational field. The aim of the lecture was to discuss how one defines angular momentum in general relativity, what the notion of intrinsic angular momentum is, how it ‘couples to a stationary gravitational field’ and whether one can measure this coupling, which turns out to be linked to the vorticity in the spacetime, by local experiments or by experiments conducted on Earth. The lecture became very catchy, mainly because the topics he covered appeared to be essential for a comprehensive understanding of some aspects of stationary spacetime geometries like frame-dragging and vorticity.

The speaker started with a brief explanation of how one deals with angular momentum in Newtonian classical mechanics and went on to generalize the same for curved space-time. He assured us that this was all lifted from box 5.6 of Misner, Thorne & Wheeler’s Gravitation.

Before moving to the derivation of the covariant equation of motion for a spinning object, he argued that the intrinsic spin of an object can only be defined with respect to the local rest frame of the body initially. This mathematically is given as,

where is just the inner product, is the spin four-vector and is the four-velocity of the spinning object along its world-line. Here, intrinsic spin is meant to describe either the <expectation of the> polarization vector of a quantum particle or the intrinsic angular momentum of a rigid body like a gyroscope or a pulsar(!?), he continued. Under the assumption that no external force acts upon the spinning object, and that its quadrupole moment doesn’t couple with inhomogeneities of the gravitational field, one naturally writes in its rest frame,

which he showed becomes covariantly,

where is the acceleration.

Prashant then (preemptively) defined the Fermi derivative of a vector along a world line , with a tangent vector as,

He went on to discuss how the notion of Fermi-transport is a useful tool in the study of spinning objects, in general relativity. The equation of motion for the spin vector reduces to the statement that the spin vector is Fermi transported along its path. i.e.,

The Fermi derivative has the property that it also Fermi transports along itself. Further, since one has and both vectors are Fermi transported (along ), the two vectors remain orthogonal all along . This implies that remains in the directions perpendicular to always and one may introduce an orthonormal spatial triad that is also orthogonal to , in which to project and its equation of motion to simplify matters.

The next discussion was on the “Frenet-Serret frame,” which happens to be just such a frame. To understand the Frenet-Serret frame clearly, Prashant restricted to defining it along an arbitrary curve in . The goal was to derive the Frenet-Serret equations, which describe how the triad fields evolve along , and to show how they involve the inherent properties of the curve like its curvature and torsion . The tangent, normal, and binormal unit vectors, denoted by , , and , form an orthonormal basis and the Frenet-Serret equations can be written in matrix form as,

Prashant then showed that the equation of motion for the spin three vector in the Frenet-Serret frame becomes just,

,

where is related to the vorticity two-form, (which vanishes in static spacetimes but is non-zero in stationary spacetimes) and the above equation is written in the FS spatial triad. This just means that a spin vector just appears to precesses in a stationary spacetime!

Prashant concluded his talk by briefly talking about some results of projects he was part of which were related to the precession of gyroscopes on Killing orbits of the Kerr spacetime. In such cases, is simply the normalised vorticity of the Killing congruence and the following table summarises some results,

He also discussed how these pertained to local experiments. We were too tired and hungry to listen to Prashant prattle on about how he’s applying this stuff to pulsars, and reading the temperature of the room correctly, he obliged us by letting us go.

To the Boundary and Back Again

Jahanur started his first lecture by explaining how to characterize gravitational radiation. Basically, he introduced a null frame and studied the complex Weyl scalars and in this frame. A detailed analysis of these Weyl scalars shows that falls off as , where is understood as affine parameter along null direction. As falls off as , it can be used to characterize the gravitational radiation.

The non orthogonal tetrad frame which we are interested is defined as , , here and are the coordinate indices which are raised and lowered by and and are the frame indices which are lowered and raised by . We can think of as a four vector defined at each point of space time. i.e, with being real and null and being null and complex. By defining along the outgoing null direction and along the incoming null direction we can choose a non orthogonal basis.

We then did the Lorentz tansformation of the tetrad frame in detail by considering three classes of rotations. The 10 component Weyl scalar has been studied under this null rotation by considering the 5 complex scalar quantities and . The first class of rotation does not change the vector and it leaves invariant. This can be shown by using the antisymmetry property of the Weyl tensor . On the other hand the second class of transformation leaves the vector unchanged as a result becomes invariant. This type of rotations are important over the others because it leaves `origin-independent’ for asymptotically flat space-time. The concept of radiation is ‘less invariant’ in cases when does not have a null character. Namely the radiative component of the field, may differ for different null geodesics approaching the same point on . An invariant characterization of gravitational radiation is no longer available in asymptotically de Sitter space-time. And the class three rotations are like scaling.

After a short coffee break, we started the second part of the lecture by reviewing the Penrose diagram for the Minkowski spacetime and then extend it to the maximally symmetric de Sitter spacetime. The spacetime can be visualized as a hyperboloid in 5 dimensional Minkowski spacetime,

and the metric has the form,

.

We then discussed certain peculiar properties of the de Sitter space-time such as not a single observer can access full de Sitter space-time. We also discussed the dependence of the global structure of the space-time on the sign of the cosmological constant and certain symmetries of the de Sitter space-time.

We then went to linearize Einstein’s equation in de Sitter backround and for that purpose we defined a trace reversed quantity, and . Gravitational source is at the origin so it is sufficient to restrict to the future Poincare patch. The metric of future Poincare patch in coordinates is conformally flat, . We make a gauge choice which will decouple the linearized equations and for further simplification the rescaled tensor with is used in the rest of the calculations. After exhausting all gauge freedoms we solved the non-zero components of field using Green’s function technique.

where,

The delta function appearing in the Green’s function corresponds to the propagation along the light cone and the theta function corresponds to the propagation inside the light cone. This is basically a generic property of the wave propagation in any curved back ground since as the wave propagates it back reacts with the background curvature and slows down.

After discussing a couple of questions Jahanur concluded the talk by explaining the idea of how to relate gravitational field with the source moments in the de Sitter background.

–
In the afternoon of the first day of second half of ST4, Chandan presented his first lecture on open quantum field theories. Open systems are those which are allowed to exchange energy with an environment. As the analysis of these theories is done in a formalism called ‘Schwinger-Keldysh,’ he spent his first lecture to cover the utility of this formalism in closed quantum field theory.

To make this point, he first went over the formalism of computing correlation functions in the interacting ground state of a closed quantum field theory. Let’s understand this. Consider an interacting quantum field theory, with a Hamiltonian where is the free Hamiltonian, and is the interacting Hamiltonian. The interacting ground state is , and let the ground state of the corresponding free theory be . By considering the time to be complex (!), we can derive an expression for the interacting ground state in terms of the free ground state.

This allows us to write the two point correlation function in the interacting ground state to correlation function in the free ground state.

where .We then need to use perturbation theory to compute the left hand side order by order in the coupling constant. However, we will need to assume for this computation, that the in vacuum and the out vacuum are related by a phase. However, this is not true in general for quantum field theories. For field theories in curved spacetime, for theories with sources, the in vacuum maybe a linear combination of the out states. Therefore to compute any such correlation function in these theories we will need to sum over all the out states. This makes the computation harder in such situations, Schwinger Keldysh formalism provides a prescription to directly calculate this sum without needing to know the out states.

What is Schwinger Keldysh formalism? How does it sum over these out states? Consider a quantum mechanical system with a Kernel which evolves a basis wave function .

where . Then the probability of the state transitioning to is given by

Chandan then showed that if we compute then we will have on the right hand side,

where is the initial density matrix. Schwinger-Keldysh formalism is in fact based on such a path integral. We can see that in this path integral, is evolved forwards in time till infinity where and then is evolved back in time.

Suppose we want to calculate the one-point function of an operator in a density matrix , we get

where we have expanded the trace in the second step. After a few manipulations we can write,

We can view this through the following time contour,

where we evolve upto time , and add an insertion at time and then evolve to and then again to . Thus, when computing one-point function in density matrices, we can obtain a way to do this via a closed time contour where we evolve forward in time and then backward evolve from infinity. We then saw how we can compute out of time ordered correlators also in this description, which are interesting in certain physical systems.

We then saw various rules in this prescription. For e. g., we can compute

provided and , with L and R denoting the reverse and forward evolving contours. Operators along R(L) are (anti-)time ordered. We then considered the possible Green’s functions in this prescription, and . In the case of the free scalar field theory, a closed quantum field theory, we computed these Green’s functions and showed that and are on-shell. We can then write diagrams for these.
–
In the evening Arpan Kundu (IMSc) gave a nice talk on soft graviton theorems and asymptotic symmetries. He began the talk by explaining how asymptotic symmetries of asymptotically flat spacetimes are related to the soft graviton theorems. Soft theorems are statements about scattering amplitudes in field theories – in a scattering process of number of external particles if momentum of any massless particle becomes infinitesimally small then the amplitude can be expressed as a product form of soft factor, which depends on momenta and polarizations of the scattered particles, and amplitude of number of finite energy particles. Soft theorems, as we understand them today, were first studied by Weinberg in sixties in the context of photon and graviton scatterings and can also be shown to hold in various scalar and gauge theories. Soft graviton theorem can be schematically expressed as

where the soft momentum scales as and the terms in the parentheses are soft factors. The soft factorization beyond leading term were discovered by Cachazo and Strominger. Based on the work by Chakrabarty, Kashyap, Sahoo, Sen and Verma and Sen, Laddha; Arpan mentioned that the soft factors, and are universal for any gravitational theory and any process and are valid to all loop levels in perturbative S-matrix provided the dimension of spacetime is greater than or equal to five. In due to the infrared divergence issues of S-matrix the expressions of the soft factors are valid to tree level only.

Next, Arpan talked about asymptotic symmetries. In the early sixties Bondi, Metzner and Sachs discovered that the asymptotic symmetry group of asymptotically flat spacetimes is not the finite dimensional Poincare group but an infinite dimensional group which is now known as BMS group. In Bondi coordinates any asymptotically flat spacetimes can be described by the following metric

The first line is the Minkowski metric and rest of the components are perturbative expansions. is the limit where one reaches the future null infinity, , which is topologically . can be mapped to complex plane whose coordinates are . It turns out that and are the free radiative data depending on and correspond to the two polarization degrees of freedom of graviton in four dimensions. Using Einstein’s equations all other metric perturbations can be solved in terms of these two data. Now the question is what are transformations that preserve the above form of the metric. These transformations are generated by supertranslations and six generators. Supertranslations are angle dependent translations at every point on and form the infinite dimensional subgroup of the BMS group and are given by the vector field

Here is any arbitrary function on depending on . Arpan explained that BMS group was extended to generalized BMS group by Alok and Miguel and where is enhanced to which contains superroation vector fields given by

where and are arbitrary vector field on .

After that Arpan went on to tell us about the recently discovered Ward identities of gravitational S-matrix by Strominger and his collaborators. Strominger conjectured that the asymptotic symmetries can be promoted to be symmetries of quantum gravity; the gravitational S-matrix is invariant under the action of the conserved charges constructed from the supertranslation and superroation vector fields at asymptotic null infinity. Since, detail derivation of these charges is time consuming, Arpan wrote down their expressions without proof. These charges contain two pieces – soft charge, which are linear in and hard charge, which are quadratic in . It is important to note that the , where is the linearized metric perturbation. Hence, the quantization of , naturally gives a quantization of free data, and hence quantization of charges. Hence, one can write the Ward identity,

 
Soft part of the charges act on the asymptotic states and insert soft graviton in the S-matrix which can be interpreted in terms of soft graviton theorems. Charges from supertranslations give rise to leading soft graviton theorem and sub-leading soft graviton theorem follows from charges from superroations.
A crucial point in this process is that, since there are two independent BMS group at and , to define symmetry of scattering process a matching condition called antipodal identifications is needed which relate free data at and antipodally on .

According to the plan, Anupam A H (IMSc) was supposed to give the second part of the talk based on their recent paper on asymptotic symmetries on double soft graviton theorems, but due to lack of time Arpan continued to briefly speak about the work. Chakrabarty, Kashyap, Sahoo, Sen and Verma derived multiple soft graviton theorem to subleading order. An interesting thing about multisoft theorems is that there can be different soft theorems based on all the soft gravitons are of same energy scale (simultaneous limit) or taken soft one after another(consecutive limit). In their paper, Anupam, Arpan and Krishnendu considered nested commutators of two generalised BMS charges with the S-matrix. They showed two supertranslation charges give rise to leading double soft graviton theorem. Leading double soft theorem is same for simultaneous and consecutive limit. At the subleading level one gets two consecutive double soft theorem apart from the simultaneous double soft theorem. The commutator evaluated between in and out scattering states produce one of the consecutive double soft graviton theorem. though formally produce the other consecutive double soft theorem, there are some mathematical subtleties about it, as the action of supertranslation charges on the superrotated vaccum is not well defined. Arpan referred us to their paper to figure out details of the story.

Blocks and Ratios, Commutants and Centres, Holographic Chaos, En Garde! We fear you no more.

Pinaki began the fourth day with a discussion on the correlation functions in a CFT and showed that the imposition of conformal symmetry is so constraining that it fixes two- and three-point functions completely, upto some constants, and , which are called the conformal data. It can also fix four-point correlators up to some arbitrary functions of conformal cross ratios. Then he went on to define what are known as conformal blocks. For this purpose, he first defined conformal partial waves (CPW).

Using an operator product expansion (OPE) he showed that a four point function can be written as,

where are conformal partial waves. Conformal blocks are functions of conformal cross ratios and related to the CPWs through a scale factor. For 2D CFTs, using conformal invariance he showed that one can set (), which simplifies the expressions of the CPWs and the CBs a lot. Pinaki also mentioned the integral representation of the CBs given by Ferrara, Grillo, Parisi and Gatto, and also warned us that computation of CBs for practical purposes wouldn’t be as simple as they seemed to appear. The forms of global conformal blocks in even spacetime dimensions are known to be given by hypergeometric functions. But Virasoro blocks in 2D are known only for special cases – there exists no closed form general expression yet.

One such case where Virasoro conformal blocks are known is the so called ‘heavy-light limit.’ In this limit, the central charge of the CFT is very large () and both the heavy () and the light operators () in the theory scale as the central charge () but they obey the following relations,

Due to the Zamolodchikovs, it is known that Virasoro conformal blocks exponentiates when as follows,

This is motivated by Liouville theory but so far there has been no honest derivation of this classical limit. Assuming this holds, and using a technique called the monodromy method, Pinaki told us that one can compute the conformal blocks, . In this way, one can show that arbitrary -point (– point) blocks with two heavy and (or ) light operators factorize into numbers of H-L-L-H blocks ( numbers of H-L-L-H blocks and one H-L-H block). This result has nice interpretation in terms of bulk geodesic diagrams in conical defect geometry (since means and one can think the dual gravity theory as being classical).

One can also use these results (particularly the one for point function) to compute twist () and anti-twist () correlator which gives entanglement entropy for disjoint intervals in exited state. The same quantity can also be reproduced from the bulk geodesic picture.

Then he went to discuss Cardy formula for 3-point coefficients, courtesy of Kraus & Maloney. It was a very natural generalisation to what he did in his third lecture with the Cardy formula, which was at the level of the partition function and therefore had no information about the three point coefficients (). To have a Cardy-like formula for three point functions he started with simplest object (containing information of ) in a CFT: one point function () at finite temperature . He defined torus 1-point function for the primary operator with dimension and with torus modular parameter . Using modular covariance,

he related behaviour with behaviour.

The ‘Cardy formula’ for the average value of OPE coefficient is given by,

where is the lowest energy state with which has non-vanishing 3-pt function.

Pinaki discussed two interesting limits.

• The so called classical limit.

and :

Again as discussed before, this has nice dual classical gravity interpretation. Operators and are light fields in the bulk, which is a BTZ black hole for large enough . And can be reproduced from the following bulk geodesic picture.

• Another (more) interesting limit when the central charge is not very large.

and :

In this case, since can be small, there is no obvious notions of classical gravity. Also, the field can have mass and can thus not be thought of a perturbative field. Therefore, one should think of as a ‘heavy’ field that back-reacts and creates a conical defect on the geometry. The deficit angle is related to the mass of – this reproduces . This is an intriguing result. It suggests that the limit is not necessary to match (some) results obtained from dual classical gravity! Another example of this of course the matching between Cardy formula for 2D CFT which is valid for and the semiclassical BTZ black hole entropy, which is strictly valid for

With these concluding comments, Pinaki bowed out on day 4 and (post coffee) Ronak came up to wrap up the lecture series on bulk reconstruction that he shared with Nirmalya.

In his last lecture, Ronak described how perturbative bulk reconstruction techniques (like HKLL) can help us construct the bulk operators that are confined to a “code subspace” of the full quantum gravity Hilbert space. We have also seen how two different HKLL representations of a bulk field need to have same action on all the states in the code subspace. That is, as long as an observer is restricted to (sufficiently) low energy experiments, he/she can not differentiate these two HKLL representations of the bulk field. The code subspace can hence be thought of as an effective field theory subspace. “In this lecture, we will see how to define a general state in the code subspace and from there we derive the Faulkner-Lewkowycz-Maldacena formula for entanglement entropy,” Ronak says.

Ronak started by introducing some fun mathematical definitions and preliminaries. An algebra is a set of operators that close under addition or multiplication. From now on, we consider algebras that are defined in the space of matrices. Also, we deal with algebras that include the identity element and that are closed under Hermitian conjugation (i.e., if ). Next, we define the notion of commutant of an algebra. For any algebra , its commutant is an algebra of all operators that commute with all the elements of . The intersection of an algebra and its commutant is called the centre of . That is, the centre commutes with all the elements of the algebra.

Suppose is an algebra of all operators on -dimensional Hilbert space, then we can always define a “density operator” such that , and , for any state in the Hilbert space, where stands for trace. Suppose is a subalgebra of and let . Then for any operator , we have . is called the reduced density operator and is uniquely fixed once the subalgebra is specified.

In the basis where all the elements of centre of subalgebra are diagonalized, all elements in the subalgebra are block diagonal and each block is characterized by their respective Casimir values. This is very much similar to how if we consider a generic state in a QFT, it can be represented as a block diagonal where each block is characterized by value. As a special case, the reduced density matrix is block diagonal and can be written as

where represent blocks.

Coming back to physics, let us divide the boundary into two different regions and . Let and be the corresponding bulk regions. Note that the code Hilbert space () can not be written as a tensor product of Hilbert spaces ( and ) of and . This can be understood by noting that and are commutants of each other and as long as their intersection is non-trivial, it is easy to see that the total Hilbert space can not be a tensor product of these two spaces. Instead, if we consider at the level of blocks, then the Hilbert space can be written as a sum of factors i.e.,

Once we have established that, we can write a general state in the code subspace as

where s are some numerical coefficients and using the subregion duality, the state can be shown to be of the following form

where the boundary Hilbert space of a block is decomposed as . Here ‘s are unitary operators acting on two boundary subspaces and . is part of the CFT Hilbert space that is not accessible to the observers in the code subspace.

The FLM formula is a step away now. We consider the reduced density matrix . Computing the von Neumann entropy corresponding to this reduced density matrix gives us the FLM formula that looks schematically as

Note that the state is responsible for the RT-term here.

This can be schematically thought of using the picture (from Harlow’s paper that this section was a summary of)

The full state in the CFT is the degrees of freedom in the two corresponding bulk regions encoded embedded in the CFT using some ‘extra entanglement’ ; the RT part of the entanglement entropy is the extra entanglement in and the bulk part is the entanglement of the bulk degrees of freedom with each other.

The lecture concluded with Ronak discussing the Jafferis-Lewkowycz-Maldacena-Suh construction and the entanglement wedge reconstruction briefly, and some of the limitations of our understanding regarding the latter. With this, another speaker bid adieu to his speaking time at ST4.

The evening talk today was offered by Kedar Kolekar (CMI, Chennai). Based on the recent interest in investigating the origin of holographic chaos in AdS/CFT and why the Lyapunov index in such theories is maximal , Kedar discusses his recent work, arguing that this further generalizes for hyperscaling violating Lifshitz theories (having a non-relativistic field theory dual).

He first briefly introduces the hyperscaling voilating Lifshitz geometry is, with a view towards generalizing the correspondence to non-relativistic holography. This involves working with hyperscaling voilating Lifshitz metric, which can be written as

which for and this reduces to the metric. The interest in such bulk theories stems from the fact that diffeomorphisms, which are the symmetry of this action, scale the time and the boundary coordinates differently. In general there can also be a horizon in the bulk,

located at the zeros of , thus introducing a temperature in the dual field theory. Of course, these do not seem to look like the solutions to vacuum Einstein’s equations and one needs to have quite an assortment of background gauge fields and matter fields which directly relate to the parameters and in this metric. The action in four dimensions is,

with

For a certain value of the two field strengths introduced above, the thermal factor takes the form

where is chosen to get the asymptotic behaviour of the metric right, while imparts electric charge . and are related to in quite a cumbersome manner.

Further, the null energy conditions on the matter fields are realized through constraints on and as,

In the extremal limit, given by , the Bekenstein–Hawking entropy turns out to be . being the transverse area of the brane.
Kedar’s goal was to show how in the near extremal limit, the near horizon dynamics is governed by a dimensionally reduced action which is the well known Jackiw–Teiteilboim action. To this end, he first shows that the extremal metric reduces to times something near the horizon. Doing a dimensional reduction yields,

where is the dilaton coming from the dimensional reduction of the metric. The metric looks like , must be related to the half dozen parameters already introduced. Also the dilaton and the matter field take a particular value.
The crucial analysis which derives the central result follows next. Given the the above background on-shell values of the metric, dilaton and the matter field , one looks at the fluctuations,

where . These fluctuations satisfy the linearized equations of motion which come from the quadratic part of the action expanded about the background . Here is the on-shell value of the action for background solution and therefore the vanishes on-shell while determines the equations of motion for the above fluctuations.
But here comes the subtelty of Jackiw-Teiteilboim theory: the boundary term inherited from the 4-dim action, i.e the Gibbons-Hawking term (see day 3 blogpost) also survives dimensional reduction and its contribution about the background solution can be similarly expanded in fluctuations. The leading order contribution therefore comes from the boundary term’s contribution to . This basically gives an action for different parametrizations of the boundary time thus describing an action for different metrics. It can be shown that this action is the Schwarzian action for the reparametrizations. The contribution coming from can be show to be subleading to that of the Schwarzian in certain special cases as the equations of motion implied by can be rearranged to be that of massive scalars.
The evening session thus came to an end and we found most of our numbers in the sparingly ventilated, bollywood music blaring mess, looking forward to our free day the following day, after a hectic four days!

 

We will be back with two fresh lecture series. The morning sessions will be on Gravitational Radiation and Charge in de Sitter Spacetime, presented by Jahanur Hoque & Aneesh P B (CMI, Chennai). The afternoon sessions will be on Open Quantum Field Theories, brought to us by Chandan Kumar Jana (ICTS, Bangalore).

Place too many Wedges on the Edge of Spacetime and Descend into Chaos!

Pinaki opened the third day of ST4, as was now usual. Waking up early in the morning is an obsolete notion to grad students and many sleepy faces bobbed around in the crowd. With most of us sipping coffee to keep ourselves awake, Pinaki commenced his lecture with an outline of things he wanted to discuss.

Pinaki started by explicating the importance of boundary conditions (as the radial coordinate goes to infinity) on fields and their radial derivatives, particularly for gauge theories like gravity. An action functional and these boundary conditions define a theory on a manifold. While varying the Einstein-Hilbert action, one obtains two pieces– the Einstein equations and the total derivative/boundary terms. In case these surface terms don’t vanish, one cannot put the on-shell action back into the partition function to obtain semi-classical physics. Therefore, to have a well defined classical limit of the theory, one needs to add a surface term to the action to begin with, such that it cancels the surface terms coming from the variation of the Einstein-Hilbert action. As a consequence, the total action will look like,

One such surface action that is generally added is the Gibbons-Hawking–York term (which roughly contains the trace of the extrinsic curvature of the boundary), which when added to the Einstein-Hilbert action makes the variational principle well defined. To hammer the point in, the reason one requires these additional boundary terms is roughly because the Einstein-Hilbert action (Ricci scalar) contains second derivatives of the metric (), and a naive variation would produce contributions from the boundary of the manifold. These surface contributions would contain not just the variation of the metric , but also of its derivative . Then, setting becomes insufficient to kill all surface terms and the variation of the GHY term exactly cancels all terms involving (see Dyer & Hinterbichler for a nice overview).

Sitting up, we realised the importance of these surface terms, particularly for theories which have no local dynamics (like the case of 3D Einstein gravity with a cosmological constant that we have been discussing). In such cases, a lot of the non-trivial dynamics comes from the boundary of these manifolds.

After this, Pinaki proceeded to explain asymptotic symmetries schematically. What are the conserved charges associated with gauge symmetries, he asked. Although we understand that gauge symmetries are redundancies in the description, he showed there could be non-trivial gauge symmetries (also known as large gauge transformations) which map one state to another physically different state and that they then become true symmetries; these are the global components of gauge symmetries. These symmetries are realized in an asymptotic sense, and one can expect to obtain conserved charges corresponding to these symmetries. One important point to remember is that these symmetries must respect the prescribed boundary conditions for fields, violating which, they are deemed forbidden. It turns out that (thanks to the seminal work by Brown-Henneaux) for asymptotically space, with Dirichlet boundary conditions, the symmetry group enhances to a Virasoro Virasoro, which is an infinite dimensional asymptotic symmetry group. This statement creates many happy faces in the audience as they are finally able to see the missing link – Virasoro algebra in which makes the equivalence of these theories to more visible than before.

Pinaki then moved to a discussion of 2D CFTs and people grew excited to see their favourite CFTs appearing on the board. After reviewing some basic facts about conformal transformations, he explained briefly how the symmetry algebra gets enhanced to an infinite dimensional one. The main focus of today’s talk shifted to two famous formulas: the Cardy formula and entropy of the BTZ black hole. To derive Cardy’s formula, first he introduced the idea of modular invariance of partition functions on a torus and used this powerful technique to work out the entropy, , which he proceeded to use to find Cardy’s formula which gives the density of asymptotic states,

and the entropy

Following this, Pinaki offered a brief historical account of work conducted around the 80’s and the 90’s. He discussed how Strominger put together these ideas and derived the entropy of a BTZ black hole, , where is the horizon of the BTZ black hole – you might remember this formula from his second lecture. He remarked that this paper was probably the most cited and important paper in theoretical high energy physics which doesn’t contain a single new equation!

With the aims of his lecture for the day achieved, Pinaki’s lecture drew to a close. At this very exciting point, where ideas from different fields were elegantly getting connected, and with the correspondence at the crossroads, fully awoken from the thrill of the lecture, we headed for lunch.

Ronak Soni (TIFR, Mumbai) started his first lecture on bulk reconstruction by providing some motivation for the famed Ryu-Takayanagi formlula. In a CFT, the entanglement entropy of a subregion with its complement is given by the von Nuemann Entropy,

where is the reduced density matrix obtained by partial tracing over complement of . Assuming the AdS-CFT correspondence, which says that in the large limit, EE can also be obtained through the Ryu-Takayanagi formula as,

where is the region on the boundary and is the surface with minimal area in the bulk and which ends on the boundary of . For this to work, the surface should not enclose any holes like a black hole. This, he pointed out, was all for time independent metrics. For the time dependent cases, one must extremise the surface.

In 2013, the next order in the expansion of the entanglement entropy was obtained. It carried the interpretation of measuring the amount of entanglement of the region enclosing the RT surface and the rest of the bulk.

Ronak then reminded us of the definition of the causal wedge (something Nirmalya spoke about the previous day) and defined for us the entanglement wedge. The causal wedge is the smallest bulk causal diamond which contains the boundary causal diamond. The entanglement wedge is the bulk causal diamond that contains both the boundary causal diamond and the RT surface. Generically, the entanglement wedge would be larger than the causal wedge, which can be seen by drawing the causal and entanglement wedges for two disconnected regions in the boundary, and . He also noted that the HKLL prescription described to us by Nirmalaya was employed causal wedges and a prescription using entanglement wedges can be constructed, but turns out to be harder.

After the short coffee break, we concerned ourselves the question ‘Which CFTs have a semiclassical bulk dual?’

• Should they necessarily have a large central charge? implies that quantum fluctuations are supressed.
• A small number of primaries (single trace and multi trace operators) maybe? With scaling dimension to create a massive bulk field, whilst avoiding back-reaction in the bulk.
• Correlators should factorise! Meaning all correlators are simply determined by the 2-point function just like a free field theory.

A problematic aspect is that such operators don’t satisfy the equations of motion. Ronak revealed to us the names of these objects– generalised free fields. They also don’t form consistent CFTs. Two ways were listed in which to see this. The stress energy tensor doesn’t exist in the multi trace spectrum and secondly, there are too many degrees of freedom which can be seen by computing the partition function for a single field. The free energy is given as,

which tells us there are more states than the volume! Ronak discussed another method to see this: a Fourier expansion to see what happens when we have equations of motion. Also, perturbation theory in also fails to reproduce the CFT result of the four-point function of these GFFs. This is important in the bulk Reconstruction context because the HKLL construction is based on the two-point factorisation assumption and then adding corrections, as was shown by Nirmalaya. This fails for arbitrary correlators. The bulk understanding of this fact is when back reaction becomes strong, the HKLL construction fails.

Ronak then introduced an application of this as a paradox in which different HKLL from different sub-regions in the boundary were used to create the same bulk operator at the same point, the resolution of which is that the HKLL prescription only holds a certain subspace of the full Hilbert Space called the code subspace, which is exactly the subspace of generalised free fields that was talked about earlier!

With this, his time was up and he promised to come back the next day for his last lecture, which also was to be the last lecture on bulk reconstruction at .

In the evening talk today, we had Avik Banerjee (SINP, Kolkata) speaking about Chaos in AdS/CFT. He began by reminding us that a classical system of particles in three dimensional space would be integrable if it possessed independent constants of motion, which also Poisson commute among themselves. He pointed out that one generally finds integrable systems for . A consequence, hence, is that most systems in nature (with a large number of degrees of freedom) tend to be chaotic, and one already begins to see inklings of why large CFTs could possibly be chaotic. “One can use the AdS/CFT duality to place a bound on the ‘amount of chaos’!,” he says. And with that preamble, we jump into the talk.

Recently, there has been a lot of interest in the Sachdev-Ye–Kitaev model since it was found that it saturates the chaos bound by Maldacena, Shenker & Stanford. The chaos bound refers to the bound on the rate of growth of chaos in thermal quantum systems with a large number of degrees of freedom. For a large CFT at finite temperature , this bound can be written as , where is the Lyaponov exponent. Via AdS/CFT, this translates to the statement that in a black hole background, gravitational perturbations exhibit maximal chaos.

de Boer et al. (2018) studied the chaotic behaviour of yet another system in the context of AdS/CFT. In the context of AdS/CFT, a heavy quark in a thermal plasma bath is dual to an open string living in a particular black brane geometry. This system is to be considered as that of a Brownian particle (quark) coupled to a (strongly interacting) thermal plasma, which was studied by de Boer et al. (2008) and the temperature of the dual CFT/plasma corresponds to the Hawking temperature of the black brane, . It turns out that considering small fluctuations due to the interactions of the quark with the thermal plasma is equivalent to studying perturbations of a static string that hangs from the boundary to the horizon, and the involvement of black holes in this story is an indicator of the possible appearance of chaos in this system.

Avik then proceeded to explain the notion of chaos starting with comments for classical systems. He quickly noted that for classical harmonic oscillator, a small change in initial conditions does not change its dynamics drastically. However, it was argued, that for chaotic systems, their dynamics are highly sensitive to initial conditions and the separation of two world-lines with slightly different initial conditions grow exponentially. Mathematically, this is,

where is the Lyaponov exponent. This is roughly the statement of classical chaos.

With de Boer et al. (2018) as a cornerstone, Avik discussed how quantum chaos is characterized by the usual prescription of upgrading poission bracket to commutator bracket, which in this case implies,

One way to analyze chaos is through the commutator between a pair of Hermitian operators. This commutator represents the sensitivity of to perturbations created at an initial time by . The strength of this effect is measured by the thermal average,

which can be broken into two pieces as,

Here, the first piece is time-ordered correlator, which is not sensitive to chaos and decays as,

where is the dissipation time. The chaotic behavior of can be probed by the out of time order correlator (OTOC),

which vanishes for a chaotic system at sufficiently large times . Since, there were some questions regarding why this should vanish at large time dynamics, Avik proceeded to offer some plausibility arguments.

There was also discussion about how the OTOC changes with time, which led us to the interesting notion of the scrambling time, (on the CFT side). Mathematically, within the time scale , the OTOC can be written as:

where is the inverse temperature and  is a positive order one constant that depends on the specific operators V and W. The time at which the second term becomes relevant gives the scrambling time as,

To make clear what a black hole has to do with chaos physically, Avik tried to depict the relevant OTOC as corresponding to some process in the bulk. The operators
V, W create two quantas in the black hole background. He finally showed
that the relevant OTOC in the CFT becomes the amplitude of two-two scattering in the
bulk, near the horizon, using a few cute pictures, courtesy of de Boer et al. arXiv: 1709.0102 (2018).

Avik proceeded to discuss developments in his recent work on the realization of a saturation of the chaos bound in a D3-D5 brane system! The schematic attack on the problem was outlined by Avik as follows:

• Find classical embeddings of such systems.
• Consider fluctuations around the embedding and solve for them.
• To find the four point function, construct the quartic action $S^{(4)}$.
• Find the overlap.

He then went on to show that even in a D5 brane worldvolume theory as
the dual theory one finds saturation of chaos bound for vector fluctuations
of D branes.

On How to Cut a Melon Without Hitting the Seeds

The beginning of the second day saw Pinaki discuss Maldacena’s decoupling argument which was employed in conjecturing the AdS/CFT duality.

To draw a parallel, Pinaki mentioned how, in QED, one describes the motion of an electron in the presence of a proton in two equivalent ways: either by treating the problem perturbatively in vacuum, using Feynman diagrams, and summing over all possible diagrams or by considering the electron to be moving in an effective potential (due to all Feynman diagrams) without the explicit presence of the proton.

Similarly, he argued, at small couplings in string theory (when strings are light objects and D-branes heavy) the motion of closed strings on the background of D-branes can be described equivalently in two ways. The first is a perturbative perspective– closed strings split into open strings on D-branes which recombine to form closed strings and leave the branes. This is described by the low energy action of an open string on the stack of branes, i.e., by the super Yang-Mills theory. In the second picture, the presence of stack of D-branes is replaced by an effective potential (due to all possible string interaction diagrams) in which the closed string moves. Since D-branes behave like a source of closed strings, due to the presence of a large number of closed string states, the stack of D-branes can be replaced by some gravitational background.

Pinaki then went on to discuss the GPKW (Gubser, Polyakov, Klebarov; Witten) master formula that relates the full quantum partition function of the CFT to the partition function of AdS gravity evaluated at the “saddle point.” This is described by the following equation

where s are dual CFT operators corresponding to the bulk fields . The non-normalisable mode of the bulk field evaluated at the boundary acts as the source for the CFT operator. To calculate the connected part of the CFT correlation functions, one has to take functional derivatives of the CFT partition function w.r.t. sources .

It was pointed out that there is a bit of subtlety involved in choosing boundary conditions when solving the bulk field equations. In the Euclidean theory, one simply demands regularity of the solution at the origin of AdS. However, in the Lorentzian computation (when calculating real time correlators), both solutions of are regular at the black hole horizon, and there is a question of choice. Son & Starinets proposed that one must pick ingoing boundary conditions at the horizon (if one is interested in the retarded correlator) while computing the on-shell action.

The main focus of the latter part of the talk was on locally spacetimes. An example with maximal symmetry is the global . Solutions can then be constructed by quotienting/orbifolding . Such solutions have fewer symmetries and can be thought of as excitations of pure AdS, though they all look the same locally. A simple example of an orbifolded geometry is a torus that can be constructed by taking the quotient of the complex plane with a lattice. Orbifolded spacetimes can have conical singularities in them that are naked singularities. These can be interpreted as alluding to the presence of some massive particle in .

Two examples of locally spacetimes are the thermal spacetime and the spacetime of the BTZ black hole. Thermal is obtained by putting the time coordinate of Euclidean on a circle. The resulting geometry is that of a solid torus. The BTZ black hole is also a solid torus. The modular parameters and of the two torii corresponding to the thermal and the BTZ black hole respectively are related by . This relation between the two torii geometries will be explicitly used to derive the Cardy formula for 2D CFTs at high temperatures and the BTZ black hole entropy later on.

Pinaki then shifted to a discussion of the first law of black hole thermodynamics in the context of the BTZ black hole. Following this, it was noted that for thermal , the entropy vanishes. Armed with some thermodynamics, we proceeded to a calculation of the free energy when Pinaki showed that above a critical temperature , the BTZ black hole partition function dominates over the thermal , with the consequence that BTZ is free-energetically preferred over thermal . For temperatures , it is the thermal AdS’ turn to dominate over BTZ. At , there is a first order phase transition due to a jump in the first derivative of the free energy. This can be visualised as an interchanging of cycles of the two torii. This phase transition is famously known as the Hawking-Page transition. Unlike black holes in flat space, the BTZ black hole has positive specific heat i.e., it’s in stable thermal equilibrium.

Pinaki’s time for the day was now up and he left us to think about the Hawking-Page transition and everything else he talked about, and on came Nirmalya to deliver his second lecture.

In his previous lecture, Nirmalya discussed the Hamilton-Kabat-Lifschytz-Lowe construction which provides a way to represent the local bulk field through CFT operators living on the boundary in the large N limit. There he implemented the HKLL construction for the free scalar field and showed that may be expressed as,

where is the “smearing function.”

Today, Nirmalya began by considering an interacting scalar field which obeys the KG equation of the form,

Here the key idea is to introduce the concept of a spacelike bulk Green’s function as,

Employing this spacelike Green’s function and the “extrapolate dictionary” one may express via,

where is a normal vector. It is to be noted that for the free scalar field theory, the second term vanishes and one recovers the results for the previous HKLL construction, as one should. Furthermore, one may iterate the previous equation to obtain a perturbative description of in terms of CFT operators . For the interacting field theory we considered above, one may now obtain the final form of the field as,

where is the smearing function. The above expansion can be represented by Feynman-like diagrams. It also tells us that we are trying to recover order by order without reproducing the bulk perturbation theory.

Nirmalya then discussed an alternative representation where the spatial support of the CFT representation is constricted to some subregion on the boundary time slices. This incorporates the concept of subregion-subregion duality, where a region in the bulk is dual to some region in the boundary. In this case, the is an embedded submanifold of with the metric,

Here and parametrize a subregion of the full which is known as Rindler-wedge. Once again the idea is to solve the KG equation in this wedge. The field in this case is expressed as,

where and are the AdS-Rindler wedge and its intersection with the AdS boundary respectively. It is important to note that in the above expression is a distribution unlike in previous cases, where is was just a function.

Finally, in the last part of his lecture, Nirmalya discussed some of the interesting features of the recent construction by Nakayama & Ooguri. Here one uses the one-to-one correspondence between the AdS isometries and CFT symmetries. The main idea here is to introduce a boundary operator which transforms under the conformal generators as a bulk field. It was shown that the isometries and keep the origin fixed in the bulk as and . Next, an ansatz was made to express the above bulk field in terms of all the descendant fields of the primaries in the CFT as,

Using the above two constraints, one may determine the coefficients . So, in this way, one may obtain the desired bulk scalar field from Nakayama-Ooguri method.

With this nice exposition on the NO method, Nirmalya bowed out. He shares his lecture series with Ronak Soni (TIFR, Mumbai) who will turn up tomorrow and pick up the baton.

Ritabrata Bhattacharya (HRI, Allahabad) offered the evening talk. He started off a discussion of the SYK Model with an overview of earlier works. Although it was orginally formulated as an interacting theory with 4 Majorana fermions, he discussed it in the context of the -fermion interaction with a Hamiltonian,

The random couplings appearing above are chosen from a probability distribution which he later declared to be Gaussian… just to make life simple. His principal aim was to evaluate the partition function for this interacting theory. This was done by integrating out i.e., the standard deviation of the couplings, followed by the introduction of a delta function which was then smeared. This is somewhat akin to the Fadeev-Popov method of quantization in the context of non-Abelian gauge theories. In the course of this evaluation, he introduced the two point correlator and eventually got to the Schwinger-Dyson equations,

where is the contribution from self-energy processes while and are the Green’s function of the free theory and the full interacting theory respectively. The most natural step after this was to connect it with the popular diagramatic representations known as ‘melonic diagrams’ (frankly, these look more like pumpkins than melons). A crucial point he highlighted was the fact that these melonic diagrams contribute because of the large limit. Analysis of the diagrammatics was followed by the exploration of the IR regime of this theory, which is probed by looking at the large limit. The central idea is that in this regime, the IR theory becomes exactly solvable and exhibits time reparametrization.

Following this introduction to SYK, Ritabrata discussed another aspect, namely the saturation of the chaos bound to the value , where is the inverse temperature. Eventually, he mentioned some recent progress he has made by introducing a chemical potential in a more generalized theory containing complex fermions. The introduction of a chemical potential, in fact, takes the theory away from an IR fixed point. As a consequence, he argued, the ‘amount of chaos is expected to go down’ due the introduction of the chemical potential, which leads to conserved charges which in turn makes the system ‘more integrable.’ Thus in some sense, the system is ‘less chaotic.’ This was explicitly shown analytically by going to the large- limit, since this theory also becomes tractable away from the conformal fixed point . In the last few minutes remaining, he provided some brief motivation and an overview of the work done by Gross & Rosenhaus.

And so, with these new perspectives on chaos from Ritabrata’s talk, it was curtains on the second day of this ever exciting workshop.

 

 

Open=Closed, Inside from Outside and Discovering Phases with Pen and Pencil

 

 

First day of ST4 2018 had some old faces, and some definitely new but the atmosphere of free inquiry was back with many people walking up to the board to explain their questions, plenty of coffee spent in the course of the three lectures in the day. Our first speaker was Pinaki, who our observant readers might remember as our first evening speaker from ST4 2017.

Pinaki’s first lecture began as he wrote the (most?) beautiful equation in theoretical high energy physics,

on the board. We all took good time to savor the beauty of this equation before he continued. Life does not provide equalities like this very often.

He then went through in brief what this equation means, why care about it and why or for these lectures. The equation above is a claim regarding the equality of certain gravitational theories to certain field theories. (AdS stands for Anti de Sitter spacetime, and CFT for Conformal Field Theory). Even though originally this was discovered (conjecturally) by Maldacena as an equality between maximally supersymmetric Yang-Mills theory in four dimensions and type IIB string theory on , we were told we will concentrate on . Why ?

1. In three dimensional (two-derivative) gravity, there are no propagating degrees of freedom. At this point there was a discussion on what this statement means. Here, various members of the audience jumped into explain different ways of showing this. Before all the explanations went half way and got jumbled up in the chaos, someone grew impatient and an explanation was offered in full. It was pointed out that in three dimensional gravity, Riemann tensor ( for ) and the Ricci tensor () have the same number of independent components. In particular, we saw that the Riemann tensor, which captures the information about the geometry, can be written in terms of the Ricci tensor, which is determined (in the case of two-derivative theories) by Einstein’s equations to be proportional to the metric.

2. Few spacetime dimensions can make life simpler. Grad students in the audience liked this point wholeheartedly.

Other simplifications were also listed before it was pointed out that the theory is still interesting despite being ‘trivial’ in this sense.

We were then told that is interesting because, unlike in higher dimensions, two dimensional CFTs enjoy infinite number of symmetry generators. We can use complex analysis for the two dimensional theory, we can use ‘modular invariance’, there exists minimal models where even without knowing the Lagrangian, one can write the correlators!

We then went through some general features of dualities, and some examples of dualities known in physics. T-duality in String theory was illustrated in some detail by considering strings wound around a compact circle direction in the manifold. It was illustrated that as the radius of the circle goes to zero, the heavy and light modes are interchanged.

After this dose of motivation and familiarization with dualities, Pinaki then introduced to us D-branes as objects where open strings end. It was reminded that open string specturm contained a gauge field, and the closed string spectrum contained a graviton (with dilaton and tensor gauge field) in their massless sector. Gauge gravity duality was motivated by how absorption and emission of closed strings by D-branes and Open strings running in loops with D-branes at their end can be dual descriptions of each other. AdS/CFT as a holographic duality was said to be further indicated by Berkenstein-Hawking entropy for black holes which scales as area.

With this set up in mind, we bravely went on to study gauge theories. t’Hooft’s double line notation was introduced briefly and we saw by examples that if we do a expansion with as the parameter of the theory, only planar diagrams appear in the leading order. Several members of the audience went upto the board before everyone was convinced that if the double line diagram rules are followed, non planar diagrams can not be written as planar diagrams.

A discussion took place on why such a limit is seen as ‘a’ classical limit. It was pointed by members of the audience that once we realize this way that is a useful way of organizing the Feynman diagrams, we can rewrite the action in terms of these parameters and see that occurs in the denominator and hence plays the same role as . i.e. one can make a saddle point approximation at large .

It was then pointed out that in string theory, the string parition function is also a sum over diagrams of different genus. We then identified and . This mapping of couplings would set the stage for the holographic dictionary to be used in the future lectures.

---

The second talk of the day was on “Bulk Reconstrution” by Nirmalya Kajuri. One of the outcomes of string theory is the celebrated conjecture about AdS/CFT duality, which Pinaki had motivated in the first lecture. According to this duality, AdS in -dimensions is dual to CFT in $d$-dimensions that lives on the boundary of AdS (also referred to as bulk).  Also, the map between various quantities on both sides is given by AdS/CFT dictionary. According to this dictionary, any bulk geometry () is mapped to a specific state in CFT (). (One simple example is that the pure AdS maps to the vacuum of the CFT.) Further, the correlators in the bulk are mapped to that of the boundary as follows:

and are bulk and boundary coordinates respectively and is the scaling dimension of the operator . is the radial coordinate of AdS and by taking the limit , we are reaching the boundary. Also, is a bulk field and is a boundary field that is related by the “extrapolate” dictionary to the bulk field as

Despite being a useful tool, this dictionary has some limitations. One such scenario where this dictionary is not as helpful is when we want to consider a bulk scattering process happening inside the horizon of a black hole. To understand such a process, we need to have a knowledge of operators that are deep inside the bulk. In these lectures, the goal would be to “construct” the operators in CFT that can represent such bulk fields. This is termed as “Bulk Reconstruction” in the literature.

Today, in the first lecture of the series, the Nirmalya dealt with the HKLL construction and then briefly discussed some potential roadblocks in this construction. Let us begin with a brief description of HKLL construction. Let is a scalar field in AdS of mass . It satisfies the Klein-Gordon equation in AdS i.e., . This equation admits the solution of the following form:

where and are the two independent plane wave solutions of the Klein-Gordon equation. Now, following the extrapolate dictionary, we impose the boundary condition: . Using the orthogonality of ‘s the solution of can be rewritten as

where the “smearing function” is given by with a similar expression for . $g_{n,l,m}$ is defined by the following relation: . Writing in this form clearly makes it non-local with respect to the CFT and also note that the smearing function is not unique. So, we have constructed a (non-local) CFT operator that represents a bulk local scalar field.

The lecture ended with an elaborate discussion on how the local observables in a diffeomorphism invariant theory do not make sense. Since the HKLL construction gives a prescription on how to construct “local” fields in the bulk, this approach should break down at some point. The speaker lastly pointed out how the free scalar field evades this problem and how the construction we have discussed makes sense.

---

Debangshu Mukherjee (IISER Bhopal) delivered the first evening lecture of this year’s ST4. The aim of his lecture was to explain the application of the gauge-gravity duality to extract the hydrodynamic behaviour of strongly coupled field theories. In particular, he demostrated the computation of shear viscosity and shear diffusion constant using the correlator of the components of the stress tensor. The dispersion relation for shear diffusion (where is the shear diffusion constant) arises as a pole in the correlation function of stress tensor components and the shear viscosity is computed by the Kubo’s formula

He began his lecture by stating the AdS/CFT correspondence and briefly motivated the study of hydrodynamics to understand quark gluon plasma (QGP) as a strongly coupled fluid. Towards understanding Kubo’s formula, he first gave a “lightning” review of linear response theory in field theory. The idea of linear response theory is to quantify the effect of perturbing a system at equilibrium as correlation functions of field theory operators which couple to the perturbations (with the perturbations acting as sources for the corresponding operators). Then to put the linear response theory in the context of gauge-gravity duality, he considered a free massive scalar field as a perturbation on background. Solving the scalar field equation , the non-normalizable mode of the solution for as we approach the boundary of acts as a source for the boundary field theory operator of dimension , which is related to mass of . Then splitting the action for into two parts: i) the bulk part vanishes by equation of motion and ii) the boundary part gives the correlation functions of s. In Einstein gravity, the kinetic term gives the two-point function of .

The shear metric perturbation couples to in the boundary field theory. The linearized Einstein’s equation for is the same as the field equation for the probe scalar . Then using the linear response theory described above, we compute the correlator and substituting it in the Kubo’s formula we get the shear viscosity . Also computing the entropy density for the black brane in , we see that the ratio of shear viscosity to entropy density is (in relativistic units), which saturates the viscosity bound proposed by Kovtun, Son and Starinets

All the above discussion pertains to relativistic field theories and their gravity duals. In last few minutes of his lecture, Debangshu showed that the viscosity bound also holds universally in a certain class of non-relativistic field theories viz., hyperscaling violating Lifshitz theories for a certain range of values of Lifshitz exponent and hyperscaling violating exponent .

—

A long day fueled by coffee (courtesy NISER and local organizers), came to an end.

P.S. See here for lecture notes and references for various lectures.

ST4 2018 at NISER Bhubaneswar ( July 6th to 14th, 2018)

 

Hi all,

We are back! Student Talks on Trending Topics in Theory (ST4) 2018 is set to happen in NISER Bhubaneswar from July 6th to 14th. The topics for the main lectures are the following:

• Assorted Topics in AdS3/CFT2 – Pinaki Banerjee (ICTS)
• Open Quantum Field Theories – Chandan Kumar Jana (ICTS)
• Bulk Reconstruction – Nirmalya Kajuri (CMI) and Ronak Soni (TIFR)
• Gravitational Radiation and Charge in de Sitter Spacetimes – Jahanur Hoque, Aneesh P B (CMI)

In addition, there will be some evening lectures as well, on each day of the meeting. We hope for a highly interactive meeting. Stay tuned for reports and updates from various lectures and discussions.

Link for the website: https://sites.google.com/view/st42018/

 

The State of Theory Comes Full Sphere

In Victor’s last talk, and the last talk of ST⁴, we turned to the computation of partition functions for gauge theories on .

The 2d chiral and vector multiplets are simply dimensional reductions of their counterparts in 4d, and they correspond respectively to matter and gauge degrees of freedom. We used the constraining power of extended supersymmetry in two dimensions to write down actions for the super-Yang-Mills and matter sectors, and noted that they are -exact. This allows us to use the localization arguments we reviewed in earlier lectures.

We are interested in solutions to the fixed-point equations (which are easy to write down as the action is written down as a sum of squares!) and when the appropriate reality conditions are imposed, we find that the scalars are required to be Cartan-valued, and further that the gauge field and auxiliary scalar are proportional to them. Dirac quantization then dictates that this gauge field flux is quantized.

Now that we have BPS solutions, we would like to compute 1-loop determinants of fluctuations about these solutions. Victor explained that there were many ways to do this, and the simplest of them is to decompose wavefunctions into spin spherical (or Wu-Yang) harmonics. In doing this, one encounters a generalized notion of spin, which is the usual spin minus a contribution coming from the quantum of flux piercing the sphere on which these gauge theories live. In the following discussions, it became clear that similar physics of flux attachment occurs when studying the quantum Hall effect.

Once the fields are decomposed into these “spin” spherical harmonics, it becomes straightforward to write down their determinant. However, when studying the 1-loop determinant of a matter multiplet for example, supersymmetry will ensure cancellations between factors contributing to the bosonic and fermionic determinants. There are, however, terms that do not cancel, and these come from chiral zero modes of the Dirac operator. Such cancellations are at the heart of any supersymmetric theory: all positive energy modes come in pairs — this follows from the SUSY algebra — but the zero modes are under no such algebraic restriction.

We played the same game with the vector multiplet and in the end, wrote down the most general partition function for a gauge theory on with matter. Victor concluded with some remarks on how the poles of the integrands that define the partition function encode information about non-perturbative sectors of the theory.

With this, all the main talks were concluded, and the audience broke into small groups that shared coffee, and boasts of who was more exhausted at the end of the workshop.

We ended a long, gruelling and satisfying two weeks with a four-hour discussion about future directions in the various sub-fields that are currently of interest in the community. We first listed out a bunch of fields and then went one by one and asked people to talk about what the interesting directions that would be worth exploring in the next five years would be. We feel safe saying that this session was extremely rewarding to all who came, helping the experts in these fields formulate what they thought was interesting, telling others what was worth expecting, and telling people who wanted to do something new what could be interesting. While we won’t try to summarise this entire discussion (for reasons of sanity), we did take a photo of the blackboard on which we listed out all the important points so that you, lovely reader, can look at it:

And, with that, we officially called to an end an amazing workshop that was our deepest honour to organise and attend.